2 4 8 16 32 64 128 256 512 1024.....for those us in the software industry, powers of 2 (exponents) are tattoo'd in our brains.
Please.... I've heard that 65568 times!
2 4 8 16 32 64 128 256 512 1024.....for those us in the software industry, powers of 2 (exponents) are tattoo'd in our brains.
i.e., 2^16 + 2^5.Please.... I've heard that 65568 times!
2^16 = 65536Please.... I've heard that 65568 times!
Do you have a cite? Because this isn't what I've been hearing anecdotally from friends in the medical community. I should say that I have read the Chinese article in Lancet, but have heard nothing official about the trials taking place in NY. Is that where your info is coming from?
Hmmm...I think my life just got better!...scholar.google.com...
There is the common usage of the word "exponential", and the mathematical one. Pretty sure the one we have been hearing refers to definition 1.
View attachment 84013
Pretty sure the one that we have been hearing refers to definition 2. This disease is growing at a rate that mathematically fits an exponential curve. And really, definition 1 is just using words to describe the curve seen in definition 2.
Sent from my iPhone using Tapatalk Pro
My point is that people use the word "exponential" in common language without regard to the actual math behind the word. "Increase becoming more and more rapid" isn't a math formula.Pretty sure the one that we have been hearing refers to definition 2. This disease is growing at a rate that mathematically fits an exponential curve. And really, definition 1 is just using words to describe the curve seen in definition 2.
Some confusion these days may stem from another common current usage. That is when people say one number is exponentially larger than another, with no implication of a change in time or with respect to an independent variable.
That one is a pet peeve of mine and just wrong in my opinion.

My point is that people use the word "exponential" in common language without regard to the actual math behind the word. "Increase becoming more and more rapid" isn't a math formula.
But these models depend on knowing how many cases there are, and testing isn't consistent across regions or even day to day. If some location hasn't been testing many people, then starts testing more, it will skew the curve and make it look like cases are increasing faster than they are. Of course it will also mean there were more cases to begin with. Garbage in, garbage out. It seems that the only way we can gauge the seriousness of the outbreak is to look at the increase in deaths due to COVID-19, or hospital admissions of people testing positive. Anecdotally, it seems that hospitals in some regions of the country and world are becoming overwhelmed. I don't think that happens during the normal flu season.You may be right regarding people’s use of the word in common language, but I assert that increasing becoming more rapid is a perfect description of the behavior of an exponential curve, and that when the news is using it, they are quoting the scientists and doctors who are well aware of the math and know that the spread of a disease in an epidemic can be well modeled with an eponential function.
Using base 2 as an example, and restricting the x values to integers, the binary exponential series is:
1, 2, 4, 8, 16, 32, 64, 128, 256, 512, 1024,...
Seems to me that sequence fits both the definitions well. Mathematically, it’s
f(x) = 2^x
And it’s also increasing at a more rapid rate.
The scary thing is that if x represents some period of time, and f(x) represents the number of people who are infected (or perhaps die from) the Covid 19 virus, then we do have a serious problem...
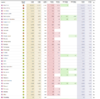
So I had a few extra minutes so I grabbed the world death data from this site:
https://www.worldometers.info/coronavirus/coronavirus-death-toll/
Plotted it and ran a "best fit" curve.
It looks like the data fits pretty well to the generic exponential equations of f(x)=ae^(kx) in the form of:
f(x)=130.05e^(0.0805x)
You assume the virus does not mutate and then take the stronger members. Or people who have been previously weekend.Sure, but it cannot continue like that. Even though it's a relatively slow growing exponent, in about 9 months, that curve kills everyone on the planet and we know that won't happen. Growing at the same rate, it kills 1% in 6 months, but it will have slowed down long before that. Every curve is going to flatten eventually because the virus will run out of weak members of the herd that succumb to it. This isn't the way to analyze it, it just leads to panic.
Reminder there is no evidence that once sick and recovered you are immune. Further complicating this mentality is there are two strains now in the "wild". As such you need to assume everyone will get it at least twice...
No, I am not aware of a trial in NY (which is not to say there aren't any, I just haven't seen them published). Always happy to provide good citations when possible.
Here is a recent one out of China - http://subject.med.wanfangdata.com.cn/UpLoad/Files/202003/43f8625d4dc74e42bbcf24795de1c77c.pdf
Here is an older one out of China for the related drug Chloroquine phosphate - https://www.jstage.jst.go.jp/article/bst/14/1/14_2020.01047/_article
Here is a study out of France which had fair controls but a small sample size - https://drive.google.com/file/d/186Bel9RqfsmEx55FDum4xY_IlWSHnGbj/view
And here is that Lancet article - https://www.thelancet.com/journals/laninf/article/PIIS1473-3099(20)30132-8/fulltext, which is really not a clinical trial but suggestions for possibly useful drugs.
To somewhat keep up on these, I search each day on scholar.google.com for 'covid-19 treatment' and restrict results to 2020. Most of interesting results in the first 3 pages. I know that a lot of physicians have apparently been prescribing hydroxyquinolone, apparently so much so as to cause a temporary shortage of it at pharmacies.
If people are interested in following this more, I post an update every day or two on my MeWe page which includes any trends in total cases in the US as well as possible treatments being developed. You can add me as a contact if already on MeWe or join and add me. mewe.com/i/petersteinmetz
But these models depend on knowing how many cases there are, and testing isn't consistent across regions or even day to day. If some location hasn't been testing many people, then starts testing more, it will skew the curve and make it look like cases are increasing faster than they are. Of course it will also mean there were more cases to begin with. Garbage in, garbage out.
It seems that the only way we can gauge the seriousness of the outbreak is to look at the increase in deaths due to COVID-19, or hospital admissions of people testing positive.
Of course. No one is saying it will continue like that. It'll flatten eventually. It'll either naturally kill everyone it was going to kill, or we do something external to bend it. Whether it's quarantining people/populations, finding a vaccine, or whatever, it'll eventually find it's inflection point and go logarithmic. The trick is trying to get it do to that sooner rather than later.Sure, but it cannot continue like that. Even though it's a relatively slow growing exponent, in about 9 months, that curve kills everyone on the planet and we know that won't happen. Growing at the same rate, it kills 1% in 6 months, but it will have slowed down long before that. Every curve is going to flatten eventually because the virus will run out of weak members of the herd that succumb to it. This isn't the way to analyze it, it just leads to panic.
Personally, I'm previously weekday.You assume the virus does not mutate and then take the stronger members. Or people who have been previously weekend....
The problem with the news...they make it sound like it’s gonns stay exponential...it’s not ...and it’s not asymptotic towards infinity. It will taper to a normal distribution.
You may be right regarding people’s use of the word in common language, but I assert that increasing becoming more rapid is a perfect description of the behavior of an exponential curve, and that when the news is using it, they are quoting the scientists and doctors who are well aware of the math and know that the spread of a disease in an epidemic can be well modeled with an eponential function.
Using base 2 as an example, and restricting the x values to integers, the binary exponential series is:
1, 2, 4, 8, 16, 32, 64, 128, 256, 512, 1024,...
Seems to me that sequence fits both the definitions well. Mathematically, it’s
f(x) = 2^x
And it’s also increasing at a more rapid rate.
The scary thing is that if x represents some period of time, and f(x) represents the number of people who are infected (or perhaps die from) the Covid 19 virus, then we do have a serious problem...
Sent from my iPhone using Tapatalk Pro
While the number of new cases per day are still quite large, the day over day new cases is slowing down. That is the rate of change is slowing. We need to see it go negative to know we are on the backside of the gaussian distribution.
Quite frankly, I'm not so sure we're not screwed anyway. We just returned from grocery shopping not 10 miles from the largest outbreak in MD to date. Though customers seemed to be willing to do their best to maintain social distancing, store management appeared to be doing nothing. Frequently touched surfaces like checkout counters and credit card terminals weren't being routinely cleaned, checkout clerks weren't gloved, one-way aisle traffic flow wasn't being directed by arrows on the floors.
Compared with the measures the Chinese put in place, what we're doing, with larger numbers of infected, seems pitifully inadequate.
A rate of new cases cannot go negative.
What we need is to get to the point where the number of new cases is exceeded by the combined number of recovered+deaths for a sustained period of time.
Huh?The problem with the news...they make it sound like it’s gonns stay exponential...it’s not ...and it’s not asymptotic towards infinity. It will taper to a normal distribution.
I spray everything with denatured alcohol. But I've done that for years. An awesome time to be an introverted germophobe.When I bring something home from the store. I bring it all to my back porch. I then take my shoes off in the garage, go inside and wash my hands, then wipe items down outside with a disinfecting wipe, and bring them inside.
Yeah, that's a decent one.Just stumbled across this on YouTube ..... very useful and thought provoking:
I spray everything with denatured alcohol. But I've done that for years. An awesome time to be an introverted germophobe.
We just had the first confirmed case in the county announced yesterday. Methinks they jumped the gun on the rural areas, we've seen nearly zero corona and about the time they inevitably have to end the shelter in place stuff is probably when we'll be in the middle of it.
Interestingly, I was just playing with the covidly dash stats and if you sort the states by infection rate per million you find California has fared well, it's in the company of more rural areas like Maine, Wisconsin, Alabama, Missouri, Montana, South Carolina.. etc. Impressive for a state that has LA and San Fran.. you would expect rates closer to New York. But it appears the swift measures taken weeks ago at the county level had a positive effectThough this was interesting
Restrictions Are Slowing Coronavirus Infections, New Data Suggest
https://apple.news/ANPrDafCtTy6-o2SzlgX67g